Coordinate geometry is a very important in 11th and 12th grade mathematics. It is also one of the largest topics in high school maths. IITJEE, BITSAT, and board exams often ask questions from coordinate geometry. Calculating asymptotes is a vital aspect of this topic and is often considered tough. For a comprehensive guide on asymptotes, read on.
We are familiar with curves like parabolae, hyperbolae, and also ellipses and circles. We know how to find tangents and normals to these curves. Let us now look at asymptotes, their significance, and how to find them.
What is an asymptote?
Some curves such as hyperbolae often tend to infinity. Lines that approach curves as they tend towards infinity are known as asymptotes. Thus, as one of the coordinates (x or y) of the curve tends to infinity, the distance between the line and curve approaches zero.
Consider the picture above. The red line is an asymptote to the curve shown in black.
Types of asymptotes
There are 3 types of asymptotes: horizontal, vertical, and also oblique. Let us learn more about them.
-
Vertical asymptotes:
A curve heads towards infinity just as x approaches some constant value c.
Here, x approaches 3 as the curves head towards infinity. Thus, x=3 can also be called the asymptote to the curve.
2. Horizontal asymptotes:
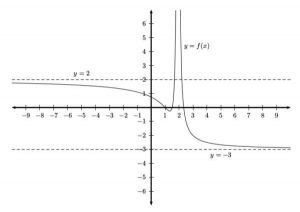
The curve approaches some constant value p as x tends to infinity.
In the above picture, we see the curve approach constant values with x tending to infinity. Thus, y=2 and y= -3 are asymptotes to the two curves at hand.
3. Oblique asymptotes
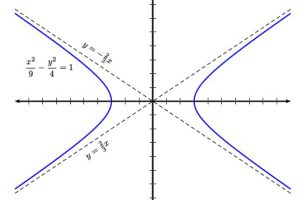
The curve approaches some line y=mx +c (m not equal to 0) while x tends to infinity.
Here, we see that the curve approaches to lines y= 2/3 x and y = -2/3 x as x tends to infinity. Thus, both those lines are asymptotes to this hyperbola.
Significance
When the graph of a curve is unknown, asymptotes play a large role in describing the nature of the graph. We can use asymptotes to sketch graphs as well. Thus, an asymptote can give us valuable information about the behaviour of curves, especially at graph edges.
An important point to note, however, is that curves can cross asymptotes.
How to calculate asymptotes
-
Horizontal asymptotes
Since we know the definition of horizontal asymptotes, we can easily find the constant c.
Let the curve be denoted by f(x). Then, if the limit of the curve where x tends to infinity or -infinity gives us c, y=c is the required asymptote.
In other words,
if lim f(x) =c
x->∞
Or
lim f(x) =c
x->-∞
is true, then y=c is the required asymptote. A curve can have a maximum of two different horizontal asymptotes.
2. Vertical asymptotes
We now know the definition of vertical asymptotes. Thus, we can also easily find the required constant p.
Let the curve be denoted by f(x). We must first solve the curve to find the domain to obtain possible constants p. Next, we check if any of the limits of f(x) where x tends to p is infinity. If so, then x=p is an asymptote.
For example, let f(x) have one solution x1.
If lim f(x) = ∞
x->x1
then x=x1 is an asymptote of the given curve.
3. Oblique asymptotes
Oblique linear asymptotes occur only if a curve approaches a line that in not parallel to either axis. Thus, let the line L(x) = mx + c be a divisor of a rational curve f(x). Then, L(x) is a candidate to be an asymptote.
Next, we check if lim [f(x) – L(x)] = 0 or lim [f(x) – L(x)] = 0.
x->∞ x->-∞
If we get lim [f(x) – L(x)] = 0 or lim [f(x) – L(x)] = 0
x->∞ x->-∞
then L(x) is an oblique and also linear asymptote of f(x).
SOLVE A PROBLEM:
Let’s give you a problem and also the steps to solve. You come up with the final answer.
Let f(x)= 3x + 7 / 2x + 5
We shall now try to find the horizontal asymptotes of the given equation.
We shall first calculate c and subsequently the line. Here, we get the domain end to be x = 5/2 because the denominator cannot be 0.
Now substitute f(x) as x tends to 5/2. Good luck completing the problem!
To find the answer to similar questions, as well as more material on asymptotes and coordinate geometry, visit edureify.com today!
